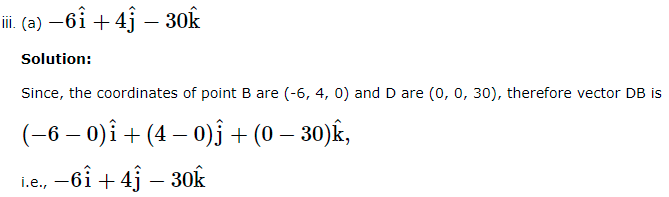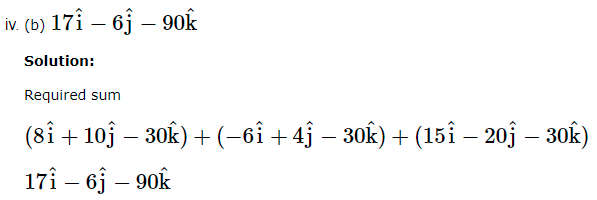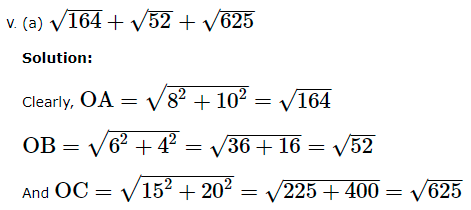Three-dimensional-Geometry
THREE-DIMENSIONAL GEOMETRY
Top Review
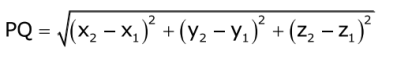
![]()


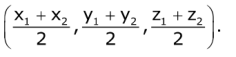
Hence, the centroid 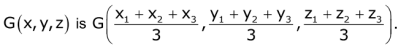
Top Concepts
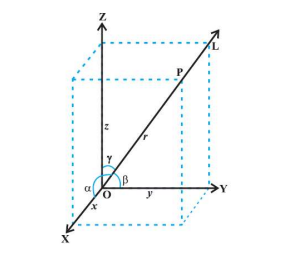
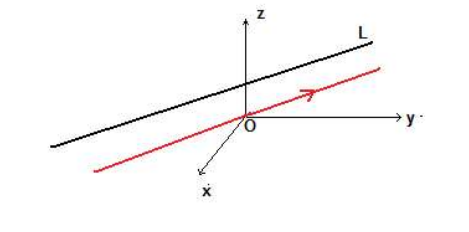
If the direction of line L is reversed, then the direction angles will ![]()
λ = cos α , m = cos β and n = cos γ are called direction cosines of line L.
x2 – x1, y2 – y1, z2 – z1 or x1 – x2, y1 – y2, z1 – z2
Similarly, the direction cosines of the y-axis are 0, 1, 0 and the z-axis are 0, 0, 1, respectively.
OR
a1b1 + a2b2 + a3b3 = 0
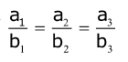
GE and DB are skew lines.
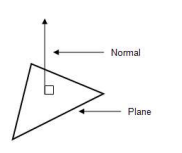
If the planes A1x+ B1y + C1z + D1 = 0 and A2x + B2y + C2z + D2 = 0 are parallel, then 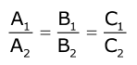
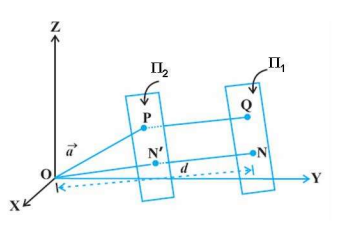
Top Formulae
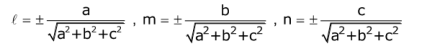
P( x1, y1, z1) and Q( x2, y2, z2) are
![]()
where ![]()
![]()
![]()
![]()
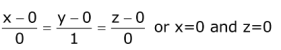
Let the Cartesian form of an equation of line be ![]()
Hence, the vector form of the equation of the line is
![]()
Let the Cartesian form of the equation of a line be
![]()
and λ is a parameter
Then the Cartesian form of the equation of the line is
![]()
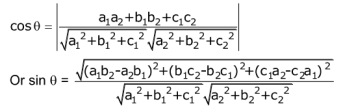
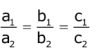
Consider the two lines:
![]()
Step (i): The general coordinates of general points on the given two lines are
(a1k + x1, b1k + y1, c1k + z1) and (a2m + x2, b2m + y2, c2m + z2)
Step (ii) Equate both the points
Thus, we have a1k + x1 = a2m + x2, b1k + y1 = b2m + y2, C1k + z1 = c2m + z2
Step (iii): Solve the first two equations to get the values of k and m. Check whether the point satisfies the third equation also. If it satisfies, then the lines intersect, otherwise they do not.
Step (iv): Substitute the values of k and m in the set of three equations to get the intersection point.
Let the two lines be

Step (i): Position vectors of arbitrary points on (1) and (2) are
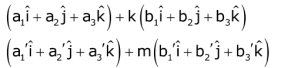
Step (ii): Because the lines (1) and (2) intersect, they intersect each other, and their points of intersection are as follows:
a1+ ka1’ = b1 + mb1’: a2 + ka2’ = b2 + mb2’; a3 + ka3’ = b3 + mb3’
Step (iii): Solve any two of the equations to get the values of k and m. Substitute the values of k and m in the third equation to check whether it satisfies it. If it does satisfy it, then the two lines intersect, otherwise they do not.
Step (iv): Substitute the values of k and m to get the point of intersection.
Let ![]() be the given line.
be the given line.
Let N be the foot of the perpendicular.
Then the coordinates of N are,
![]()
Now, the distance PN can be determined using the distance formula.
Step (i): Let P(→u) be the given point. Let →r – →a + k→b be the position vector of the line.
Step (ii): Find −−→PN – Position vector of N — Position vector of P
Step (iii): −−→PN. →b = 0
Step (iv): Get the value of k
Step (v): Substitute the value of k in →r – →a + k→b
Step (vi): Compute |−−→PN| to obtain the perpendicular distance.
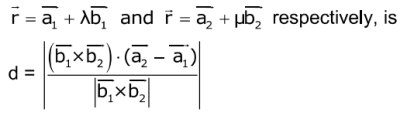
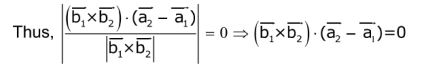
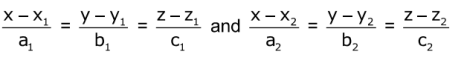
is given by
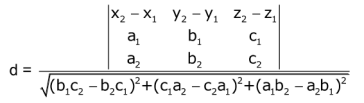
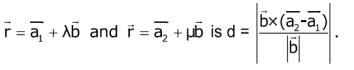
Let ![]()
Then the Cartesian equation of a plane is,
![]()
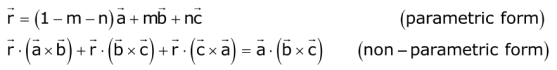
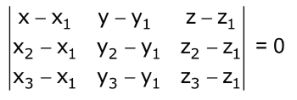

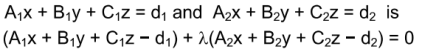
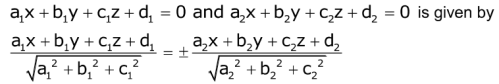
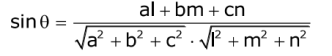
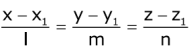 lies in the plane ax + by + cz + d = 0 if
lies in the plane ax + by + cz + d = 0 if![]()
![]()
![]()

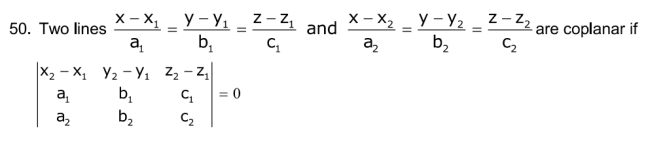
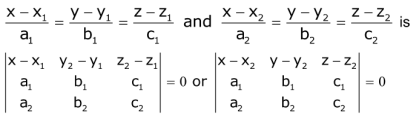

The direction ratios of the normal to the planes are A1, B1, C1 and A2, B2, C2.
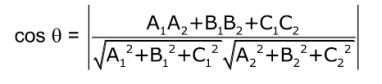
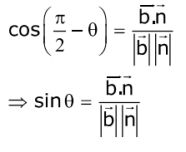
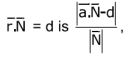 where →N is the normal to the plane.
where →N is the normal to the plane.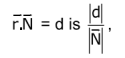 where →N is the normal to the plane.
where →N is the normal to the plane.Important Questions
Multiple Choice questions-
1. Distance between two planes:
2x + 3y + 4z = 5 and 4x + 6y + 8z = 12 is
(a) 2 units
(b) 4 units
(c) 8 units
(d) 1√29 units.
2. The planes 2x – y + 4z = 3 and 5x – 2.5y +10 z = 6 are
(a) perpendicular
(b) parallel
(c) intersect along y-axis
(d) passes through (0, 0, 54)
3. The co-ordinates of the foot of the perpendicular drawn from the point (2, 5, 7) on the x-axis are given by:
(a) (2, 0, 0)
(b) (0, 5, 0)
(c) (0, 0, 7)
(d) (0, 5, 7).
4. If α, ß, γ are the angles that a line makes with the positive direction of x, y, z axis, respectively, then the direction-cosines of the line are:
(a) < sin α, sin ß, sin γ >
(b) < cos α, cos ß, cos γ >
(c) < tan α, tan ß, tan γ >
(d) < cos² α, cos² ß, cos² γ >.
5. The distance of a point P(a, b, c) from x-axis is
(a) √a2+c2
(b) √a2+b2
(c) √b2+c2
(d) b² + c².
6. If the direction-cosines of a line are < k, k, k >, then
(a) k > 0
(b) 0 < k < 1
(c) k = 1
(d) k = 1√3 or – 1√3
7. The reflection of the point (α, ß, γ) in the xy-plane is:
(a) (α, ß, 0)
(b) (0, 0, γ)
(c) (-α, -ß, γ)
(d) (α, ß, -γ).
8. What is the distance (in units) between two planes:
3x + 5y + 7z = 3 and 9x + 15y + 21z = 9?
(a) 0
(b) 3
(c) 6√83
(d) 6.
9. The equation of the line in vector form passing through the point (-1, 3, 5) and parallel to line x−32 = y−43, z = 2 is
(a) →r = (-ˆi + 3ˆj + 5ˆk) + λ(2ˆi +3ˆj + ˆk)
(b)→r = (-ˆi+ 3ˆj + 5ˆk) + λ(2ˆi + 3ˆj)
(c) →r = (2ˆi+ 3ˆj– 2ˆk) + λ(-ˆi + 3ˆj + 5ˆk)
(d) →r = (2ˆi + 3ˆj) + λ(-ˆi + 3ˆj + 5ˆk).
10. Let the line x−23 = y−1−5 = z−22 lie in the plane x + 3y – αz + ß = 0. Then (α, ß) equals:
(a) (-6, -17)
(b) (5, -15)ss
(c) (-5, 5)
(d) (6, -17).
Very Short Questions:
![]()
Short Questions:
< 1,1,2 > and <-3, -4,1 >.
and
![]()
and check whether the lines are parallel or perpendicular. (C.B.S.E. 2011)
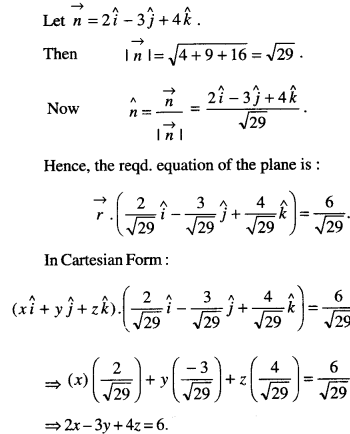
units from the origin and its normal vector from the origin is 2ˆi−3ˆj+4ˆk. Also, find its cartesian form. (N.C.E.R.T.)
![]()
→r=(ˆi−ˆj+ˆk)+λ(2ˆi−ˆj+3ˆk) and the plane →r⋅(2ˆi+ˆj−ˆk)=4 Also, find whether the line is parallel to the plane or not .
1−x3=7y−14λ=z−32 and 7−7x3λ=y−51=6−z5 are at right angles. Also, find whether the lines are intersecting or not
Long Questions:
cos2 α + cos2 β + cos2 γ + cos2 δ= 43. (N.C.E.R.T.)
x+12=1−y4=z+21
Hence, find the shortest distance between the lines. (C.B.S.E. Sample Paper 2018-19)
Case Study Questions:
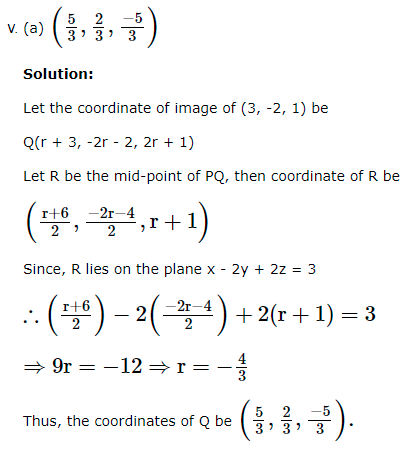
1.Suppose the floor of a hotel is made up of mirror polished Kota stone. Also, there is a large crystal chandelier attached at the ceiling of the hotel. Consider the floor of the hotel as a plane having equation x – 2y + 2z = 3 and crystal chandelier at the point (3, -2, 1).

Based on the above information, answer the following questions.
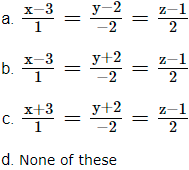
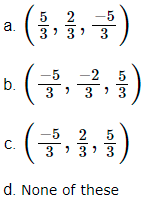
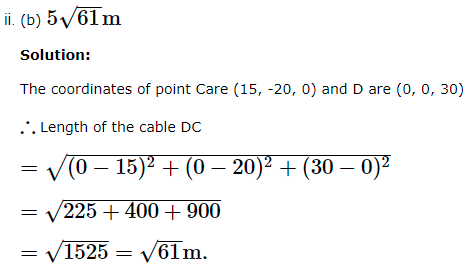
2.Consider the following diagram, where the forces in the cable are given.

Based on the above information, answer the following questions.

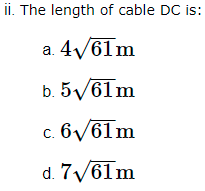

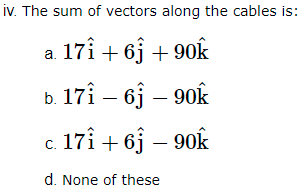
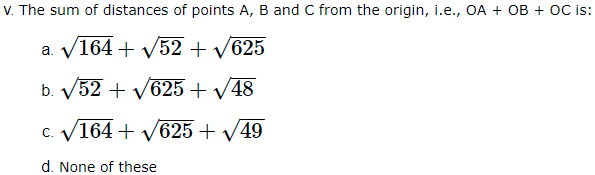
Answer Key-
Multiple Choice questions-
Very Short Answer:
l2 + m2 + n2 = 1
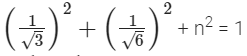
![]()
n2 = 1 – 12
n2 = 12
n = 1√2
Thus, cos α = 1√2
Hence, α = 45° or π4
![]()
Its direction-ratios are <2,-1,2>.
Hence, its direction- cosine are:

Since α, β, γ are direction-angles of a line,
∴ cos2 α + cos2 β + cos2γ = 1
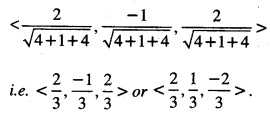
⇒ 1 + cos2α + 1 + cos2β + 1 + cos2γ = 2
⇒ cos 2α + cos 2β + cos 2γ + 1 = 0, which is true.
The given plane is2x + y – z = 5
![]()
Its intercepts are x5/2, 5 and -5.
Hence, the length of the intercept on the x-axis is x5/2
Solution:
Length of the perpendicular from P(3, -4,5) on the z-axis
![]()
![]()
![]()

Direction cosines of the line are:
< cos 90°, cos 135°, cos 45° >
![]()
The equations of the line through A (3,4,1) and B (5,1,6) are:
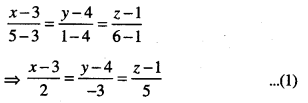
Any point on (1) is (3 + 2k,4- 3k, 1 + 5k) …. (2)
This lies on xy-plane (z = 0).
∴ 1 + 5k = 0 ⇒ k = −15
Putting in (2), [ 3-25, 4 +35, 1-1)
i.e. (135, 235, 0)
which are the reqd. co-ordinates of the point.
The vector equation of the line is →r =→a+λ→m
i.e., →r =(3ˆi+4ˆj+5ˆk)+λ(2ˆi+2ˆj−3ˆk)
Short Answer:
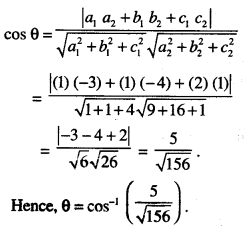
The given lines can be rewritten as:
![]()
![]()
Here < 2,7, – 3 > and < -1,2,4 > are direction- ratios of lines (1) and (2) respectively.
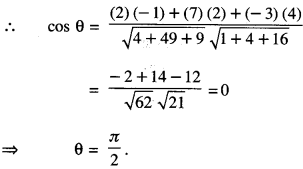
Hence, the given lines are perpendicular.
Vector equation of the line passing through
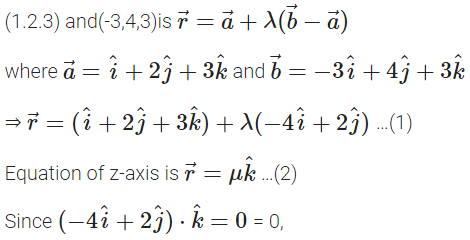
∴ Line (1) is perpendicular to z-axis.

Dividing (1) by 7,

Vector in the direction of first line
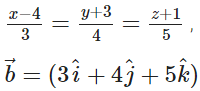
Vector in the direction of second line
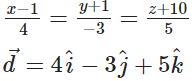
∴θ, the angle between two given lines is given by:

The given line is:
![]()
![]()
![]()
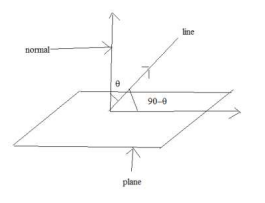
If ‘θ’ is the angle between the line and the plane,
![]()
Then
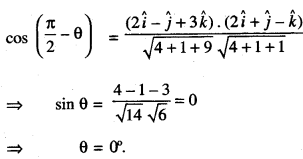
Hence, the line is parallel to the plane.
(i) The given lines are
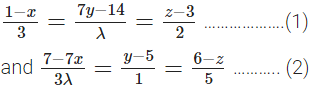
These are perpendicular if:
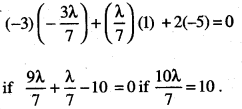
Hence λ = 1.
(ii) The direction cosines ofline(1) are <-3,1,2>
The direction cosines of line (2) are < -3,1, -5 >
Clearly, the lines are intersecting.
Long Answer:
Comparing given equations with:
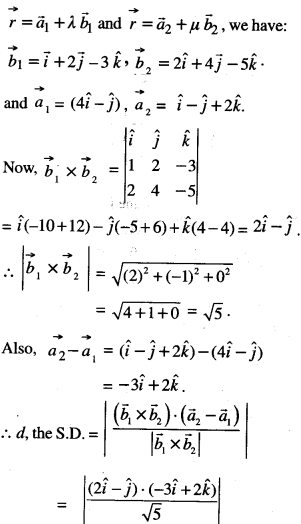
Let O be the origin and OA, OB, OC (each = a) be the axes.
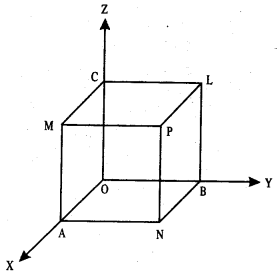
Thus the co-ordinates of the points are :
O (0,0,0), A (a, 0,0), B (0, a, 0), C (0,0, a),
P (a, a, a), L (0, a, a), M (a, 0, a), N (a, a, 0).
Here OP, AL, BM and CN are four diagonals.
Let < l, m, n > be the direction-cosines of the given line.
Now direction-ratios of OP are:
<a-0,a-0,a-0>i.e.<a,a,a>
i.e. < 1,1,1 >,
direction-ratios of AL are:
<0-a, a-0, a-0> i.e. <-a,a,a>
i.e. <-l, 1,1 >,
direction-ratios of BM are:
<a-0,0-a, a-0>
i.e. <a,-a,a> i.e. < 1,-1, 1 >
and direction-ratios of CN are:
<a-0,a-0,0-a> i.e. <a,a,-a>
i.e. < 1,1,-1 >.
Thus the direction-cosines of OP are:
![]()
the direction-cosines of AL are:
![]()
the direction-cosines of BM are:
![]()
and the direction-cosines of CN are:
![]()
If the given line makes an angle ‘a’ with OP, then:
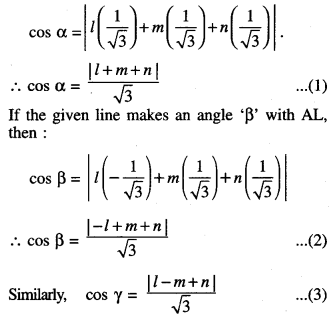

Squaring and adding (1), (2), (3) and (4), we get:
cos2 α + cos2 β + cos2 γ + cos2δ
![]()
+ (l-m + n)2 + (l + m — n)2]
![]()
![]()
The two given lines are:
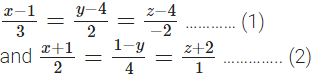
Let <a, b, c> be the direction-ratios of the normal to the plane containing line (1).
∴ Equation of the plane is:
a(x- l) + b(y-4) + c(z-4) …(3),
where 3a + 2b – 2c = 0 …(4)
[∵ Reqd. plane contains line (1)] and 2a – 4b + 1.c = 0
[∵ line (1) a parallel to the reqd. plane] Solving (4) and (5),
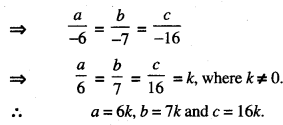
Putting in (3),
6k(x- 1) + 7k(y – 4) + 16k(z – 4) = 0
= 6(x – 1) + 7(y – 4) + 16(z – 4) =0
[∵k ≠ 0]
⇒ 6x + 7y+ 16z-98 = 0,
which is the required equation of the plane.
Now, S.D. between two lines = perpendicular distance of (-1,1, – 2) from the plane
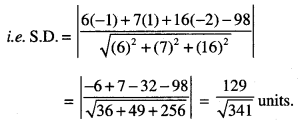
6(–1) + 7(1) +16(-2) – 98
V(6)2+(7)2+(16)2
-6 + 7-32-98 V36 + 49 + 256
(i) Cartesian equations
Any plane through (2,2, -1) is:
a(x – 2) + b(y- 2) + c(z + 1) = 0 … (1)
Since the plane passes through the points (3,4,2) and (7,0,6),
∴ a(3 – 2) + b(4 – 2) + c(2 +1) = 0
and a(7 – 2) + b(0 – 2) + c(6 + 1) = 0
⇒ a + 2b + 3c = 0 …(2)
and 5a – 2b + 7c = 0 …(3)
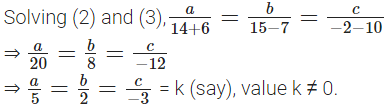
∴ a = 5k,b = 2k and c = -3k,
Putting the values of a, b, c in (1), we get:
5k(x – 2) + 2k(y – 2) – 3k(z + 1) = 0
⇒ 5(x-2) + 2(y-2)-3(z+ 1) =0[∵ k ≠ 0]
=» 5x- 10 + 2y-4-3z-3 = 0
=» 5x + 2y-3z-17 = 0, …(4)
which is the reqd. Cartesian equation.
![]()
(ii) Any plane parallel to (4) is
5x + 2y – 3z + λ – 0 … (5)
Since it passes through (4, 3,1),
5(4) + 2(3) – 3(1) + λ = 0
⇒ 20 + 6 — 3 + λ = 0
⇒λ = -23.
Putting in (5), 5x + 2y – 3z – 23 = 0, which is the reqd. equation.
![]()
Case Study Answers:
1. Answer :

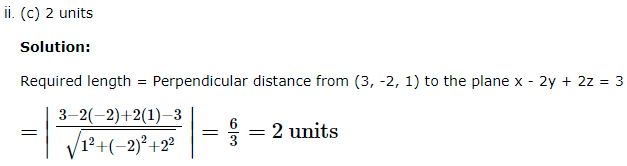

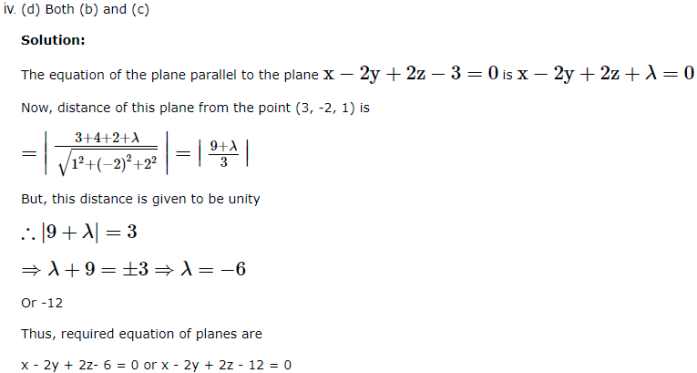
2. Answer :