
Integrals
INTEGRALS
Top Concepts
Family of curves representing the integral of 3x2

∫f(x)dx = F(x)+ C, represents a family of curves where different values of C correspond to different members of the family, and these members are obtained by shifting any one of the curves parallel to itself.
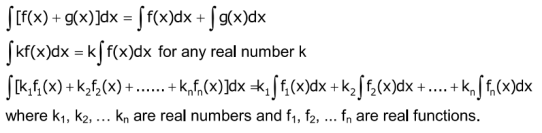
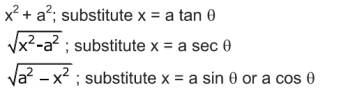
A rational function P(x)Q(x)can be expressed as the sum of partial fractions if P(x)Q(x). This takes any of the forms:

I – inverse trigonometric
L – logarithmic
A – algebraic
T – trigonometric
E – exponential is used to identify the first function.
Integral of the product of two functions = (first function) × (integral of the second function) – integral of [(differential coefficient of the first function) × (integral of the second function)].



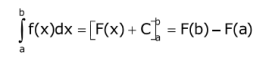
Top Formulae


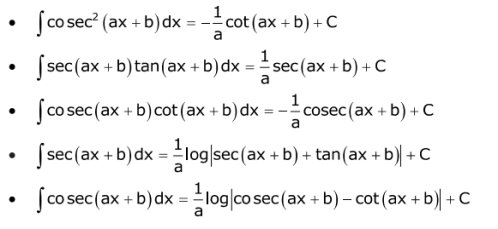
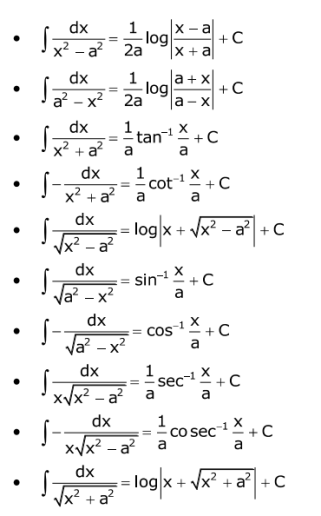
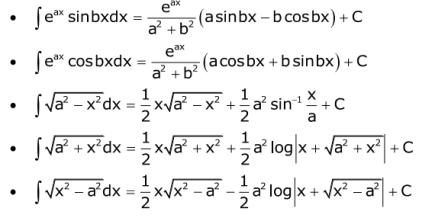
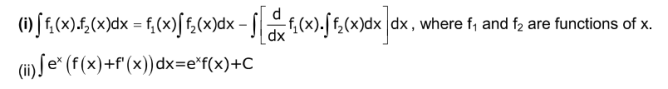
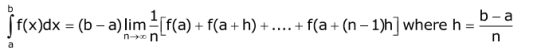
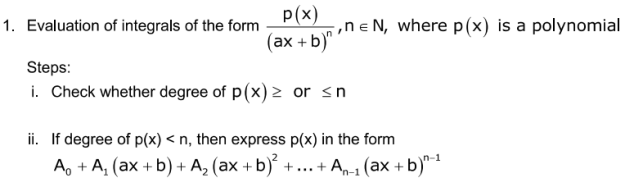
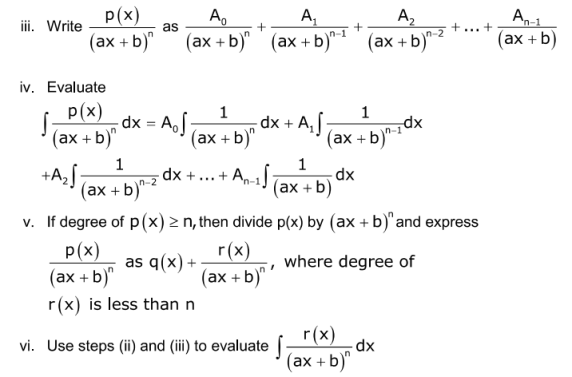
Step:
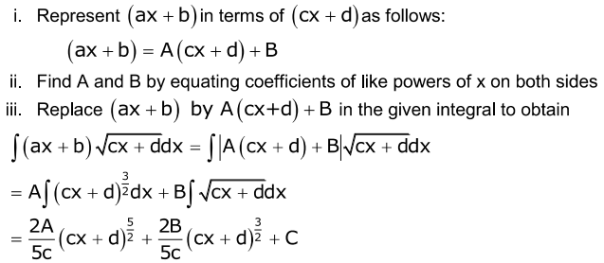
Step:
(ax + b) = A (cx + d) + B

Let us express sinm x and cosmx in terms of sines and cosines of multiples of x by using the following identities:
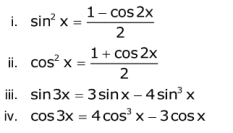
∫sinmx.cosnxdx, ∫sin mx .sinnxdx, ∫cos mx.cosnxdx
Let us use the following identities:
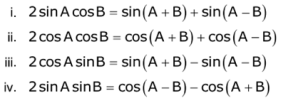
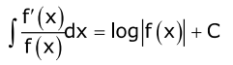
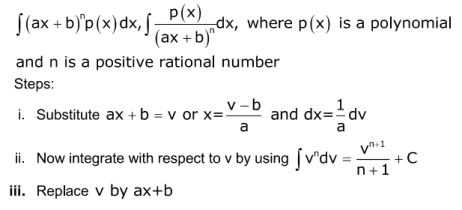


Steps:
If the exponent of cosx is an odd positive integer, then put sinx = v
If the exponents of both sinx and cosx are odd positive integers, then put either sinx = v or cosx = v
If the exponents of both sinx and cosx are even positive integers, then rewrite sinm x cosn x in terms of sines and cosines of multiples of x by using trigonometric results.

Steps:
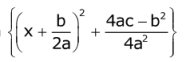
Steps:
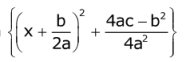
Steps:

Steps:

Steps:
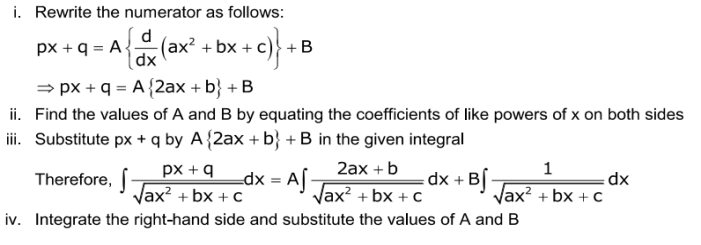

Steps:
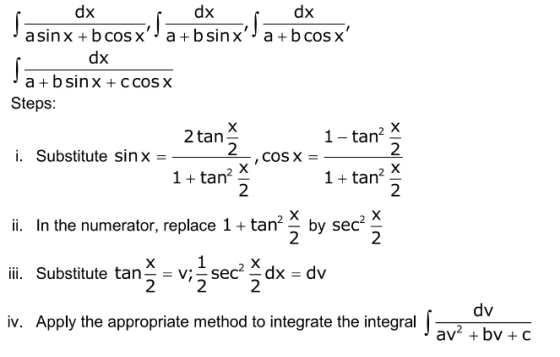
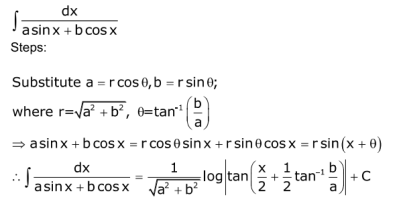

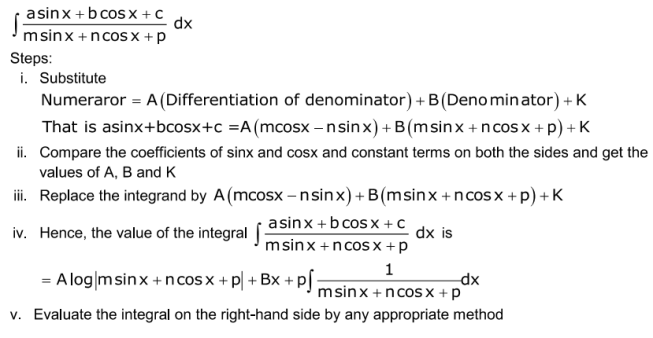



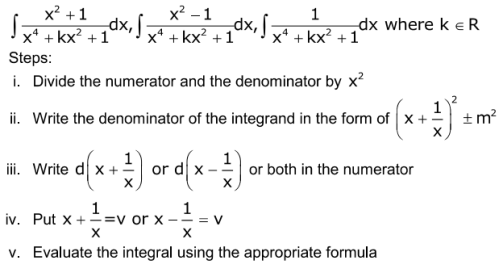
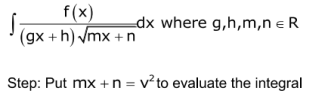
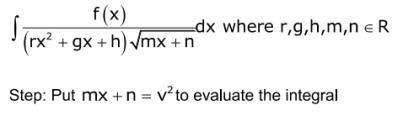

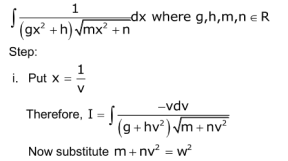
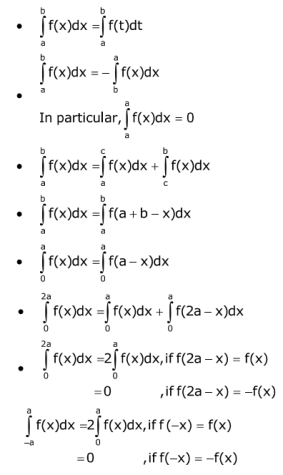
Important Questions
Multiple Choice questions-
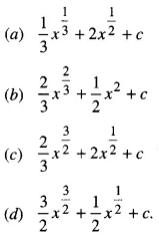
2. If 1dx (f(x)) = 4x³ – 3x4 such that f(2) = 0 then f(x) is ……………
![]()
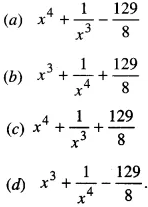
3.
(a) 10x – x10 + c
(b) 10x + x10 + c
(c) (10x – x10)-1 + c
![]() (d) log (10x + x10) + c.
(d) log (10x + x10) + c.
4.
(a) tan x + cot x + c
(b) tan x – cot x + c
(c) tan x cot x + c
![]() (d) tan x – cot 2x + c.
(d) tan x – cot 2x + c.
5.
(a) tan x + cot x + c
(b) tan x + cosec x + c
(c) -tan x + cot x + c
![]() (d) tan x + sec x + c.
(d) tan x + sec x + c.
6.
(a) -cot (xex) + c
(b) tan (xex) + c
(c) tan (ex) + c
![]() (d) cot (ex) + c
(d) cot (ex) + c
7.
(a) x tan-1 (x + 1) + c
(b) tan-1 (x + 1) + c
(c) (x + 1) tan-1 x + c
![]() (d) tan-1 x + c.
(d) tan-1 x + c.
8.
![]()
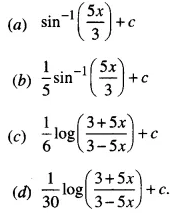
9.
![]()
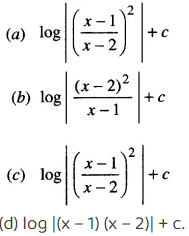
10.
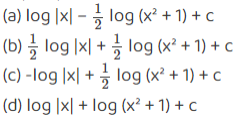
Very Short Questions:
Short Questions:
![]()
Long Questions:
Case Study Questions-
1. Integration is the process of finding the antiderivative of a function. In this process, we are provided with the derivative of a function and asked to find out the function (i.e., Primitive) Integration is the inverse process of differentiation.
Let f (x) be a function of x. If there is a function g(x), such that d/dx (g(x)) = f (x), then g(x) is called an integral of f (x) w.r.t x and is denoted by ∫f (x )dx = g(x) + c, where c is constant of integration.
(i) ∫(3x+4)3 dx is equal to:

(ii)![]()
![]()
(iii)∫sin2(x) dx is equal to:
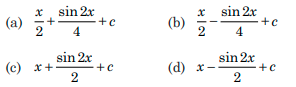
(iv) ∫tan2(x) dx is equal to:
![]()
(v) ![]()
![]()
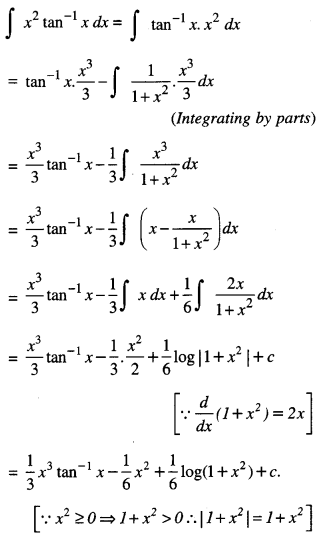
2. When the integrated can be expressed as a product of two functions, one of which can be differentiated and the other can be integrated, then we apply integration by parts. If f(x) = first function (that can be differentiated) and g(x) = second function (that can be integrated), then the preference of this order can be decided by the word “ILATE”, where
I stands for Inverse Trigonometric Function
L stands for Logarithmic Function
A stands for Algebraic Function
T stands for Trigonometric Function
E stands for Exponential Function, then
![]()
(i) ∫x.sin3x dx =

(ii) ∫ log(x + 1) dx =

(iii) ![]()
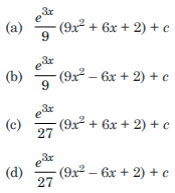
(iv) ![]()
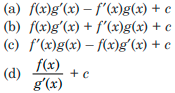
Answer Key-
Multiple Choice questions-
Very Short Answer:
I = ∫ 3+3cos xx+sinx dx = 3 log lx + sin xl + c.
[∵ Num. = ddx denom.]
I = ∫cos 4x dx = sin 4x4 + c
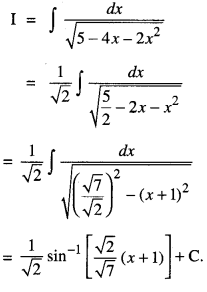


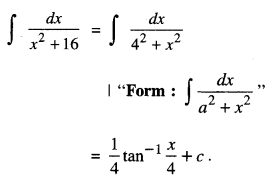
I = ∫2−2x3 dx + ∫2−21⋅dx = I1
![]()
⇒ 2 – (-2) = 4.
∫π/20ex (sin x – cos x)dx
∫π/20ex (-cos x + sin x)dx
I” Form: ∫ex (f(x) + f'(x) dx”
![]()
= -e π/2 cos π2 + e0 cos 0
= -e π/2 (0) + (1) (1)
= -0 + 1 = 1
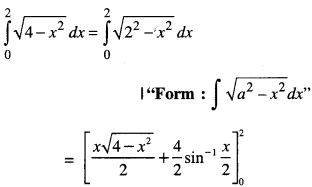
= [0 + 2 sin-1(1)] – [0 + 0]
= 2sin-1(1) = 2(π/2) = π
We have: f(x) = ∫x0t sin t dt.
f'(x) = x sin x. ddx (x) – 0
[Property XII; Leibnitz’s Rule]
= x sin x . (1)
= x sin x.
Short Answer:
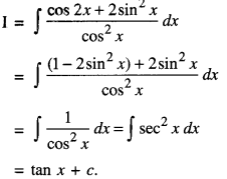
I = sec2 x√tan2 x + 4
Put tan x = t so that sec2 x dx = dt.
∴ I = ∫ dt√t2+22
= log |t + √t2+4| + C
= log |tan x + √t2+4| + C
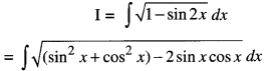
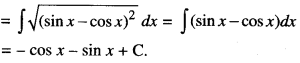
∫sinx . log cos x dx
Put cox x = t
so that – sin x dx = dt
i.e., sin x dx = – dt.
∴ I = –∫log t.1dt
= -[ log t.t – ∫ 1/t. t dt ]
[Integrating by parts]
= – [t log t – t] + C = f(1 – log t) + C
= cos x (1 – log (cos x)) + C.
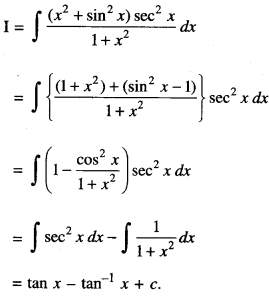
![]()
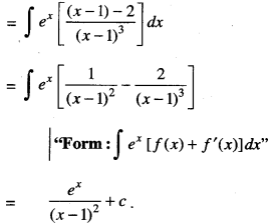
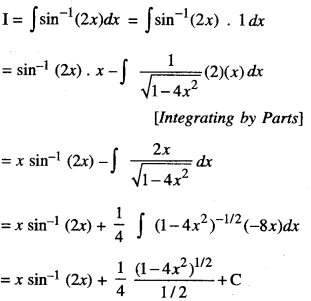
![]()
Here, f(x) = (1 – x2) sin x cos2 x.
f(x) = (1 – x2) sin (-x) cos2 (-x)
= – (1 – x2) sin x cos2 x
= -f(x)
⇒ f is an odd function.
Hence, I = 0.
Long Answer:
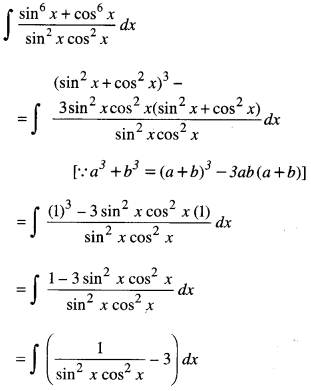
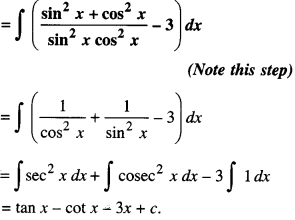

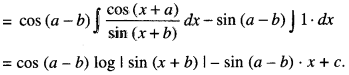
Let ∫ [log (log x) + 1(logx)2 ] dx
= ∫ log (log x) dx + ∫ 1(logx)2 ] dx …… (1)
Let I = I1 + I2
Now I1 = ∫ log (log x) dx
=∫ log (log x) 1 dx
= log (log x).x – ∫ 1logx⋅x x.dx
(Integrating by parts)
= xlog(logx) – ∫ 1logx dx ……….. (2)
Let I1 = I3 + I4
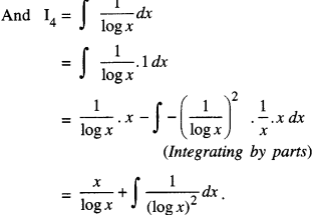
Putting in (2),
![]()
Putting in (1),
I = x log (log x)

Case Study Answers-
1.
(i) ![]()
(ii) ![]()
(iii) ![]()
(iv) (d) tan x – x + c
(v) ![]()
2.
(i) ![]()
(ii) ![]()
(iii) ![]()
(iv) ![]()






