Applications-of-Trigonometry
Some Application of Trigonometry
Ratio of the sides of a right triangle with respect to the acute angles is called the trigonometric ratios of the angle.
Trigonometric ratios of acute angle A in right triangle ABC are given below:
The values of the trigonometric ratios of an angle do not vary with the length of the sides of the triangle, if the angles remain the same.
The ratios cosec A, sec A and cot A are the reciprocals of the ratios sin A, cos A and tan A respectively as given:
Two angles are said to complementary angles if their sum is equal to 90o. Based on this relation, the trigonometric ratios of complementary angles are given as follows:
Note: tan 0o = 0 = cot 90o, sec 0o = 1 = cosec 90o, sec 90o, cosec 0o, tan 90o and cot 0o are not defined.
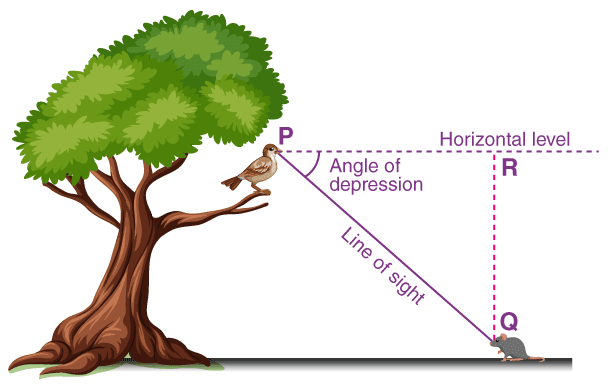
The line of sight is the line drawn from the eye of an observer to the point in the object viewed by the observer.
It states that “In a right triangle, square of the hypotenuse is equal to the sum of the square of the other two sides”.
When any two sides of a right triangle are given, its third side can be obtained by using Pythagoras theorem.
In case of reflection from the water surface, the two heights above and below the ground level are equal in length.
|
∠A |
0° |
30° |
45° |
60° |
90° |
|
sin A |
0 |
12 |
1√2 |
√32 |
1 |
|
cos A |
1 |
√32 |
1√2 |
12 |
0 |
|
tan A |
0 |
1√3 |
1 |
√3 |
Not defined |
|
cosec A |
Not defined |
2 |
√2 |
2√3 |
1 |
|
sec A |
1 |
2√3 |
√2 |
2 |
Not defined |
|
cot A |
Not defined |
√3 |
1 |
1√3 |
0 |
Horizontal Level and Line of Sight
Line of sight and horizontal level
Line of sight is the line drawn from the eye of the observer to the point on the object viewed by the observer.
Horizontal level is the horizontal line through the eye of the observer.
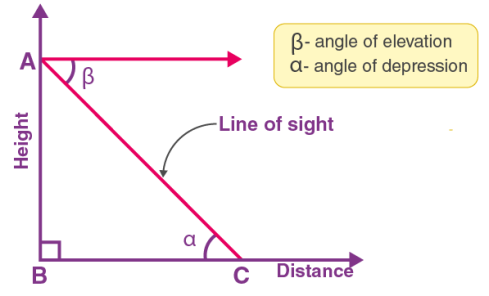
Angle of elevation
The angle of elevation is relevant for objects above horizontal level.
It is the angle formed by the line of sight with the horizontal level.
Angle of depression
The angle of depression is relevant for objects below horizontal level.
It is the angle formed by the line of sight with the horizontal level.
To, calculate heights and distances, we can make use of trigonometric ratios.
Step 1: Draw a line diagram corresponding to the problem.
Step 2: Mark all known heights, distances and angles and denote unknown lengths by variables.
Step 3: Use the values of various trigonometric ratios of the angles to obtain the unknown lengths from the known lengths.
Height and Distance in Trigonometry
The measurement of an object facing vertically is the height. Distance is defined as the measurement of an object from a point in a horizontal direction. If an imaginary line is drawn from the observation point to the top edge of the object, a triangle is formed by the vertical, horizontal and imaginary line.
From the figure, the point of observation is C. AB denotes the object’s height. BC gives the distance between the object and the observer. The line of sight is given by AC. Angles alpha and beta represent the angle of elevation and depression respectively. If any of the two quantities are provided [a side or an angle], the remaining can be found using them. The law of alternate angles states that the magnitude of the angle of elevation and angle of depression are equal in magnitude. tan α = height / distance
Large distances can be measured by the parallax method. The parallax angle is half the angle between two line of sights when an object is viewed from two different positions. Knowing the parallax angle and the distance between the two positions, large distances can be measured.
Solved Examples
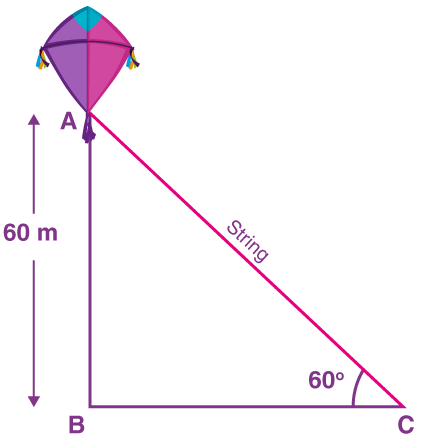
Example 1: A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in the string.
Solution:
Let A be the position of a kite at a height of 60 m above the ground.
Thus, AB = 60 m
Also, AC is the length of the string.
Angle of inclination = ∠ACB = 60
In right triangle ABC,
sin 60° = AB/AC
√3/2 = 60/AC
AC = (60 × 2)√/3
= (120 × √3)/(√3 × √3)
= (120√3)/3
= 40√3
Therefore, the length of the string is 40√3 m.
Example 2: A TV tower stands vertically on a bank of a canal. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From another point 20 m away from this point on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30° as shown in the figure. Find the height of the tower and the width of the canal.
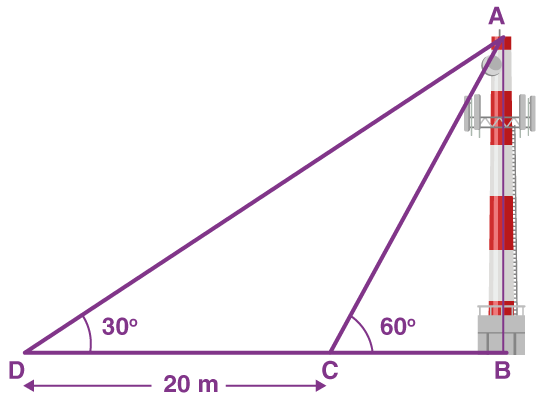
Solution:
Given,
AB is the height of the tower.
DC = 20 m (given)
In right ΔABD,
tan 30° = AB/BD
1/√3 = AB/(20 + BC)
AB = (20 + BC)/√3….(i)
In right ΔABC,
tan 60° = AB/BC
√3 = AB/BC
AB = √3 BC….(ii)
From (i) and (ii),
√3 BC = (20 + BC)/√3
3 BC = 20 + BC
2 BC = 20
BC = 10
Substituting the value of BC in equation (ii),
AB = (20 + 10)/√3 = 30/√3 = 10√3
Therefore, the height of the tower is 10√3 m and the width of the canal is 10 m.
Important Questions
Multiple Choice questions-
1. The tops of two poles of height 16m and 10m are connected by a wire. If the wire makes an angle of 60° with the horizontal, then the length of the wire is
(a) 10m
(b) 12m
(c) 16m
(d) 18m
2. A 20 m long ladder touches the wall at a height of 10 m. The angle which the ladder makes with the horizontal is
(a) 450
(b) 300
(c) 900
(d) 600
3. If the length of the shadow of a tower is √3 times that of its height, then the angle of elevation of the sun is
(a) 30°
(b) 45°
(c) 60°
(d) 75°
4. If sun’s elevation is 60° then a pole of height 6 m will cast a shadow of length
(a) 3√2 m
(b) 6√3 m
(c) 2√3 m
(d) √3 m
5. The angle of elevation of top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30°. The length of the tower is
(a) √3 m
(b) 2√3 m
(c) 5√3m
(d) 10√3 m
6. A contractor planned to install a slide for the children to play in a park. If he prefers to have a slide whose top is at a height of 1.5m and is inclined at an angle of 30° to the ground, then the length of the slide would be
(a) 1.5m
(b) 2√3m
(c) √3m
(d) 3m
7. When the length of shadow of a vertical pole is equal to √3 times of its height, the angle of elevation of the Sun’s altitude is
(a) 30°
(b) 45°
(c) 60°
(d) 15
8. From a point P on the level ground, the angle of elevation of the top of a tower is 30°. If the tower is 100m high, the distance between P and the foot of the tower is
(a) 100√3m
(b) 200√3m
(c) 300√3m
(d) 150√3m
9. When the sun’s altitude changes from 30° to 60°, the length of the shadow of a tower decreases by 70m. What is the height of the tower?
(a) 35 m
(b) 140 m
(c) 60.6 m
(d) 20.2 m
10. The ___________ of an object is the angle formed by the line of sight with the horizontal when the object is below the horizontal level.
(a) line of sight
(b) angle of elevation
(c) angle of depression
(d) none of these
Very Short Questions:

Short Questions :
Long Questions :
OR
From the top of a 7 m high building, the angle of elevation of the top of a tower is 60° and the angle of depression of its foot is 45°. Find the height of the tower. (Use √3 = 1.732]
Case Study Answers:
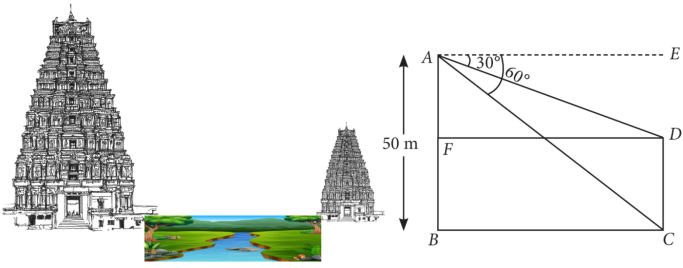
Based on the above information, answer the following questions.
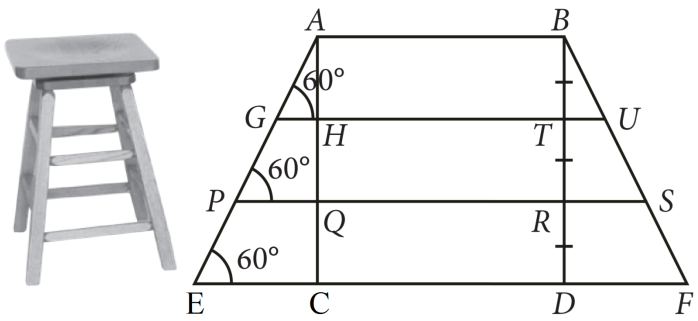
Based on the above information, answer the following questions. Take √3=1.73
Assertion Reason Questions-
Assertion: In the figure, if BC = 20 m, then height AB is 11.56 m
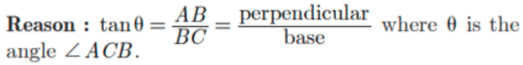
Assertion: If the length of shadow of a vertical pole is equal to its height, then the angle of elevation of the sun is 450
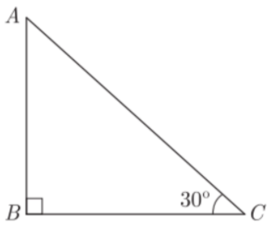
Reason: According to pythagoras theorem, h2 = 12 + b2, where h = hypotenuse, 1 = length and b = base.
Answer Key-
Multiple Choice questions-
Very Short Answer :
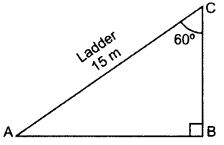
∠BAC = 180° – 90° – 60o = 30°
sin 30° = BCAC
12 = BC15
2BC = 15
BC = 152m

θ = ?
In ∆ABC,

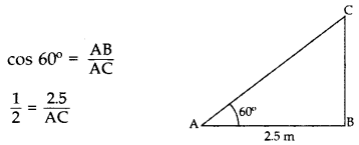
∴ Length of ladder, AC = 5 m 2.5 m
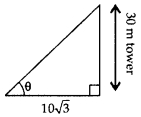
tan θ = 3010√3
tan θ = √3
⇒ tan θ = tan 60° ∴ θ = 60°
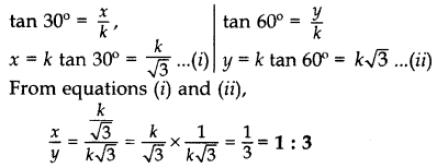
Let AB be the tower
Then, ∠C = 45°, AB = 12 m
![]()
∴ The length of the shadow is 12 m.
In right ∆ABC, we have
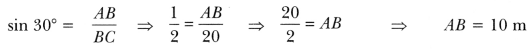
Therefore, height of the pole is 10 m.
Short Answer :
Now, in right angle ∆CBA we have,
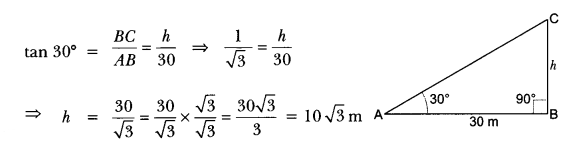
Hence, the height of the tower is 10 √3 m.
So, the total height of tree = (AB + AC)
Now in right angle ∆ABC,
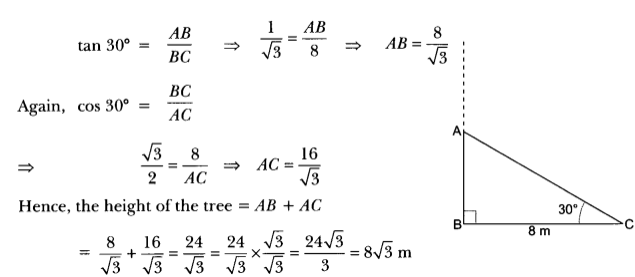
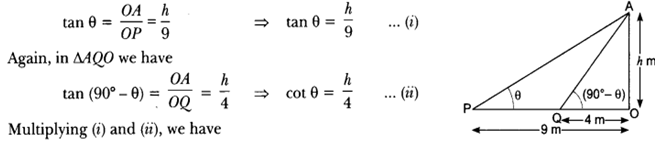
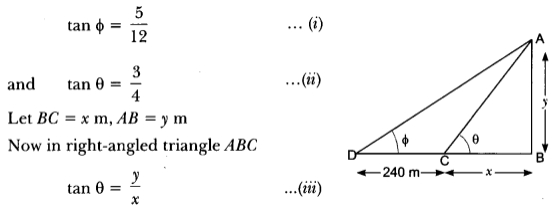
Let OA be the tower of height h meter and P, l be the two points at distance of 9 m and 4 m respectively from the base of the tower.
Now, we have OP = 9 m, OQ = 4 m,
Let ∠APO = θ, ∠AQO = (90° – θ)
and OA = h meter (Fig. 11.21)
Now, in ∆POA, we have
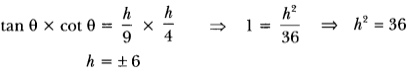
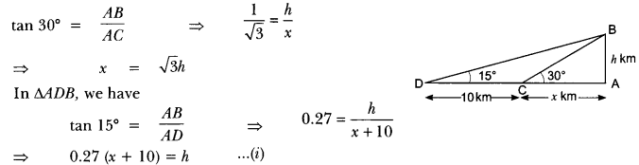
Height cannot be negative.
Hence, the height of the tower is 6 meter.
In MBC, we have
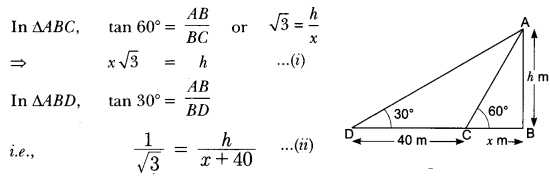
Substituting x = √3h in equation (i), we get
⇒ 0.27 ( √3h + 10) = h
= 0.27 × √3h + 0.27 × 10 = h
⇒ 2.7 = h – 0.27 × √3h
⇒ 27 = h (1 – 0.27 × √3)
⇒ 27 = h (1 – 0.46)
⇒ h = 2.70.54 = 5
Hence, the height of the mountain is 5 km
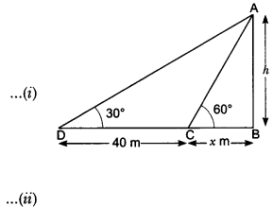
In Fig. AB is the tower and BC is the length of the shadow when the Sun’s altitude is 60°, i.e., the angle of elevation of the top of the tower from the tip of the shadow is 60° and DB is the length of the shadow, when the angle of elevation is 30°.
Now, let AB be h m and BC be x m.
According to the question, DB is 40 m longer than BC.
So, BD = (40 + x) m
Now, we have two right triangles ABC and ABD.

Using (i) in (ii), we get (x √3 ) √3 = x + 40, i.e., 3x = x + 40
i.e., x = 20
So, h = 20 √3 [From (i)]
Therefore, the height of the tower is 20 √3 m.

In Fig. AB denotes the height of the building, BD the flagstaff and P the given point. Note that there are two right triangles PAB and PAD. We are required to find the length of the flagstaff, i.e., BD and the distance of the building from the point P, i.e., PA.
Since, we know the height of the building AB, we will first consider the right ∆PAB.
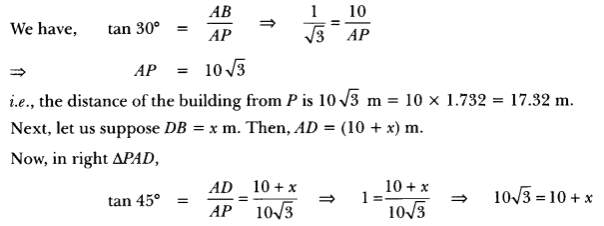
i.e., x = 100(√3 – 1) = 7.32
So, the length of the flagstaff is 7.32 m.
Now, in right angle ∆DBE, we have
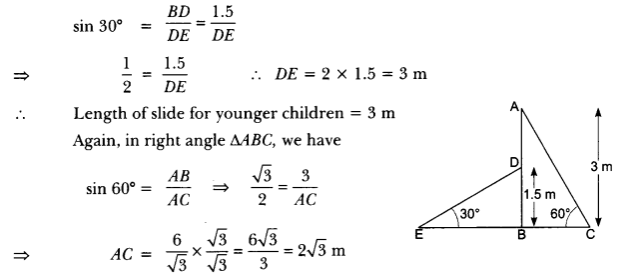
So, the length of slide for elder children is 2 √3 m.
∠KAB = 60°
Now, in right angle ∆ABK we have
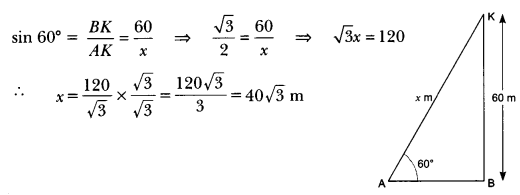
So, the length of string is 40 √3 m.
∠APR = 30°
and AB = 30 m
Now, let the new position of the boy be P’Q’ at a distance QQ’.
Here, ∠AP’R = 60°
Now, in ∆ARP, we have

Therefore, required distance, QQ = PP’ = PR – P’R
= 28.5 √3 – 9.5 √3 = 19√3
Hence, distance walked by the boy is 19√3 m.
In right ∆ADP, ∠A = 30°

∴ DB = 3m
Now, AB = BD + AD = 3 + 3 √3 = 3 (1 + √3) m
Therefore, the width of the river is 3(√3 + 1) m.
Long Answer :
Here, ∠BDA = 45° and ∠ADC = 60°
Now, in ∆ADC, we have
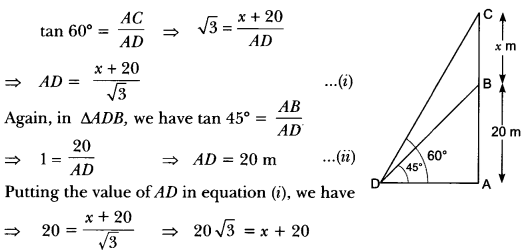
⇒ x = 20√3 – 20 = 20 (√3 – 1) = 20 (1.732 – 1) = 20 × 0.732 = 14.64 m
Hence, the height of tower is 14.64 m.
Let D be any point on the ground such that,
∠BDA = 45° and ∠CDA = 60°
Now, in ∆BDA, we have
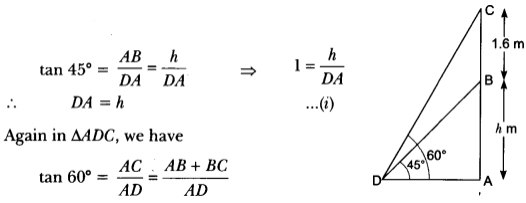
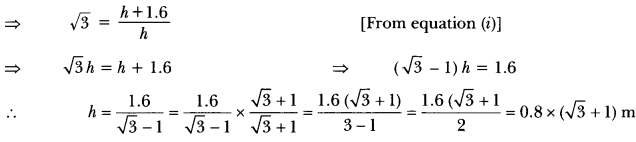
Hence, height of the pedestal is 0.8 (√3 + 1) m.
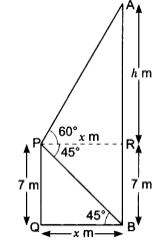
Let PQ be the building of height 7 metres and AB be the cable tower. Now it is given that the angle of elevation of the top A of the tower observed from the top P of building is 60° and the angle of depression of the base B of the tower observed from P is 45° (Fig. 11.38).
So, ∠APR = 60° and ∠QBP = 45°
Let QB = x m, AR = h m then, PR = x m
Now, in ∆APR, we have
tan 60° = ARPR
⇒ √3 = hx
⇒ √3x = h
⇒ h = √3x ……(i)
Again, in ∆PBQ we have
tan 45˚ = PQQB
⇒ 1 = 7x
⇒ x = 7 ……(ii)
Putting the value of x in equation (i), we have
h = √3 × 7 = 7 √3
i.e., AR = 7 √3 metres
So, the height of tower = AB = AR + RB = 7 √3 + 7 = 7(√3 + 1) m.

Hence, the height of the tower is 225 metres.
Now, we have
AC = BD = BQ – DQ = 88.2 m – 1.2 m = 87 m .
∠AGC = 60°, ∠BGD = 30°
Now, in ∆AGC, we have

Hence, the balloon travels 58 √3 metres.
After 6 seconds, the car reaches to such that the angle of depression at Q is 60°. Let the speed of the car be v metre per second. Then,
PQ = 6υ (∵ Distance = speed × time)
and let the car take t seconds to reach the tower OA from Q (Fig. 11.41). Then, OQ = υt metres.
Now, in ∆AQO, we have
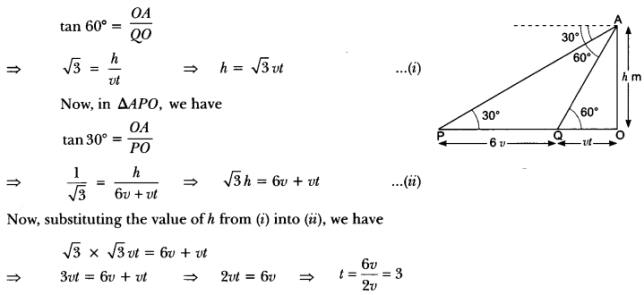
Hence, the car will reach the tower from Q in 3 seconds.
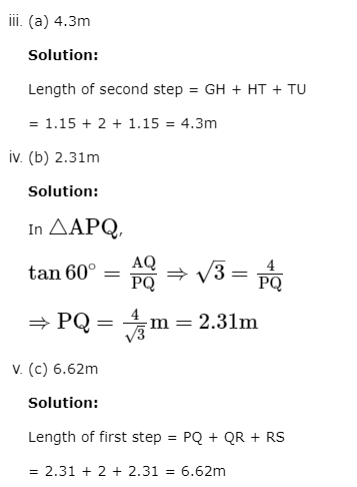
AP = 1.8 m
AJ = JK = KP = 0.6 m
AK = 2AJ = 1.2 m
In ∆ARJ and ∆BNJ’ we have
AJ = BJ, ∠ARJ = ∠BNJ = 60°
and ∠AJR = ∠BJ’N = 90°
∴ ∆ARJ ≅ ∆BNJ
⇒ RJ = NJ (By AAS congruence criterion)
Similarly, ∆ALK ≅ ∆BMK”
⇒ LK = MK”
In ∆ARJ,
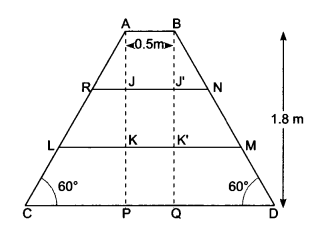
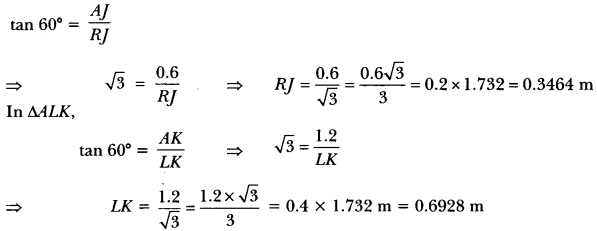
Since ∆ACP ≅ ∆BDQ
So, BD = AC = 2.0784 m
Now, RN = RJ + JJ + J’N
= 2RJ + AB [∵ RJ = J’ N and JJ = AB]
= 2 × 0.3464 +0.5 = 1.1928 m
Length of step LM = LK + KK + KM
= 2LK + AB [∵ LK = K M and KK = AB]
= 2 × 0.6928 + 0.5 = 1.8856 m
Thus, length of each leg = 2.0784 m = 2.1 m
Length of step RN = 1.1928 m = 1.2 m
and, length of step LM = 1.8856 m = 1.9 m
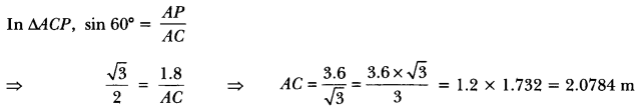
Let AB and CD be two poles of equal height h metre and let P be any point between the poles, such that
∠APB = 60° and ∠DPC = 30°
The distance between two poles is 80m.(Given)
Let AP = x m, then PC = (80 – x) m.
h’m Now, in ∆APB, we have
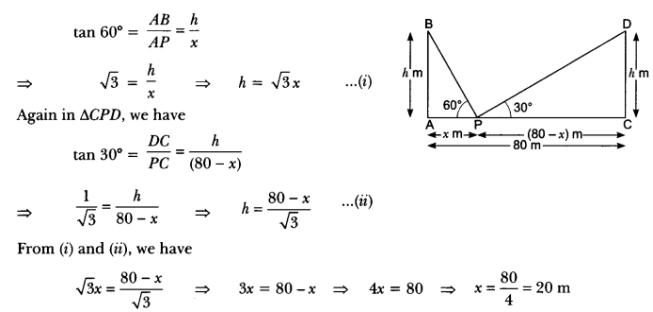
Now, putting the value of x in equation (i), we have
h = √3 × 20 = 20 √3
Hence, the height of the pole is 20 √3 m and the distance of the point from first pole is 20 m and that of the second pole is 60 m.
Now in ∆ABC, we have
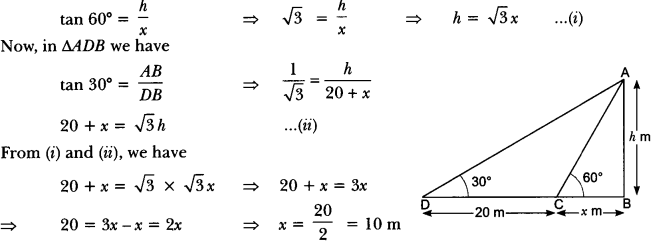
Now, putting the value of x in equation (i), we have
h = √3 × 10 = 10√3
⇒ h = 10 73 m
Hence, height of the tower is 10 √3 m and width of the canal is 10 m.
∠ACB = 60° and ∠ADB = 30°.
In ∆ACB, we have
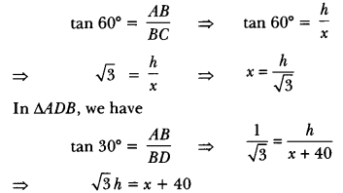
![]()
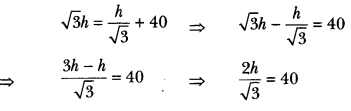
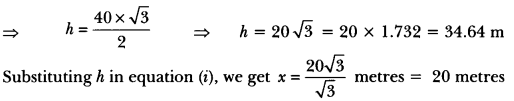
Hence, the height of the tree is 34.64 m and width of the river is 20 m.
Case Study Answers:
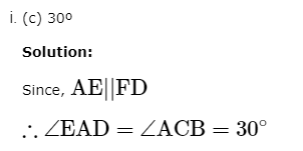


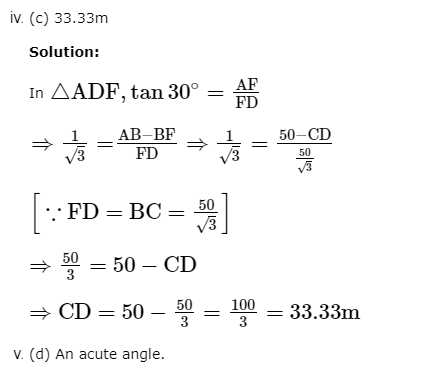
Given, side of square top = 2m
∴ Given, side of square top = 2m
Also, AC and BD are perpendicular to the ground.Also,
So, AH= HQ = QC (By B.P.T. Theorem)

Assertion Reason Answer-
(a) Both A and R are true and R is the correct explanation of A.
(b) Both A and R are true and R is not the correct explanation of A.