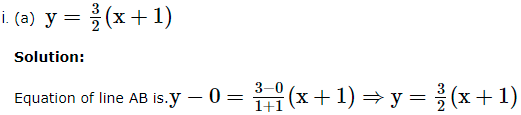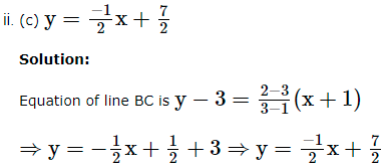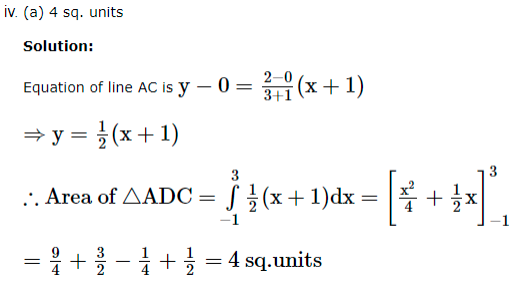Applications-of-Integrals
APPLICATION OF INTEGRALS
Area=∫ba[f(x)−g(x)]dx+∫bc[g(x)−f(x)]dx
Important Questions
Multiple Choice questions-
1. Area lying in the first quadrant and bounded by the circle x² + y² = 4 and the lines x = 0 and x = 2 is
(a) π
(b) π2
(c) π3
(d) π4
2. Area of the region bounded by the curve y² = 4x, y-axis and the line y = 3 is
(a) 2
(b) 94
(c) 93
(d) 92
3. Smaller area enclosed by the circle x² + y² = 4 and the line x + y = 2 is
(a) 2 (π – 2)
(b) π – 2
(c) 2π – 1
(d) 2 (π + 2).
4. Area lying between the curves y² = 4x and y = 2 is:
(a) 23
(b) 13
(c) 14
(d) 34
5. Area bounded by the curve y = x³, the x-axis and the ordinates x = -2 and x = 1 is
(a) -9
(b) – 154
(c) 154
(d) 174
6. The area bounded by the curve y = x|x|, x-axis and the ordinates x = -1 and x = 1 is given by
(a) 0
(b) – 13
(c) 23
(d) 43
7. The area of the circle x² + y² = 16 exterior to the parabola y² = 6x is
(a) 43 (4π – √3)
(b) 13 (4π + √3)
(c) 23 (8π – √3)
(d) 43 (8π + √3)
8. The area enclosed by the circle x² + y² = 2 is equal to
(a) 4π sq. units
(b) 2√2 π sq. units
(c) 4π² sq. units
(d) 2π sq. units.
9. The area enclosed by the ellipse x2a2 + y2b2 = 1 is equal to
(a) π²ab
(b) πab
(c) πa²b
(d) πab².
10. The area of the region bounded by the curve y = x² and the line y = 16 is
(a) 323
(b) 2563
(c) 643
(d) 1283
Very Short Questions:
x = π3
{(x,y): x2 + y2 ≤ 1 ≤ x + 4}
Long Questions:
x2 + y2 = a2. (N.C.E.R.T.)
{(x,y): x2 + y2< 8, x2< 2y}. (C.B.S.E. Sample Paper 2018-19)
Case Study Questions:
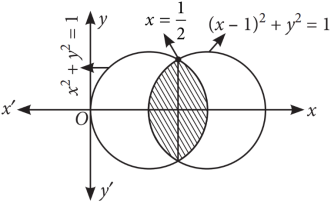
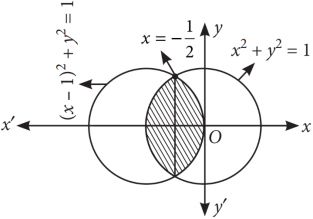
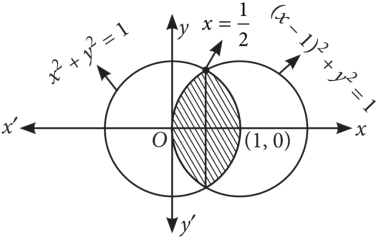
1. Ajay cut two circular pieces of cardboard and placed one upon other as shown in figure. One of the circle represents the equation (x – 1)2 + y2 = 1, while other circle represents the equation x2 + y2 = 1.
Based on the above information, answer the following questions.
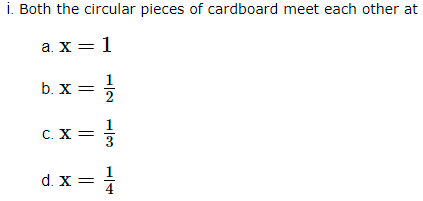
![]()

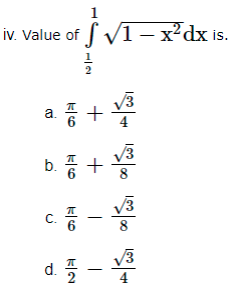
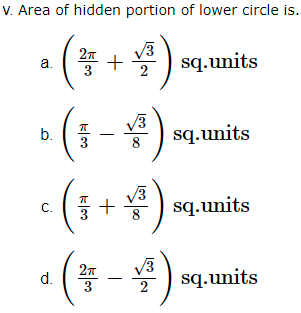
2. Location of three houses of a society is represented by the points A(-1, 0), B(1, 3) and C(3, 2) as shown in figure.
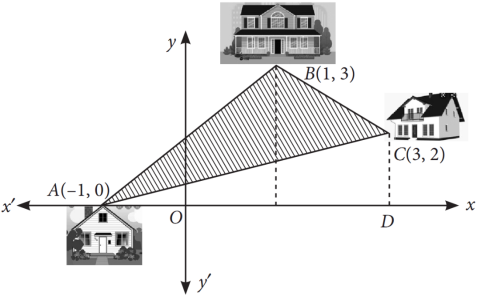
Based on the above information, answer the following questions
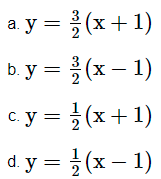
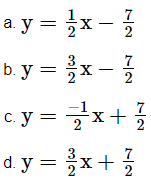
Answer Key-
Multiple Choice questions-
Very Short Answer:
Long Answer:
The given circle is
x2 + y2 = a2 …………. (1)
This is a circle whose center is (0,0) and radius ‘a’.

Area of the circle=4 x (area of the region OABO, bounded by the curve, x-axis and ordinates x = 0, x = a)
[ ∵ Circle is symmetrical about both the axes]
= 4 ∫a0ydx [Taking vertical strips] o
![]()
![]()
But region OABO lies in 1st quadrant, ∴ y is + ve]

We have:
y = x …(l)
and x2 + y2 = 32 …(2)
(1) is a st. line, passing through (0,0) and (2) is a circle with centre (0,0) and radius 4√2 units. Solving (1) and (2) :
Putting the value of y from (1) in (2), we get:
x2 + x2 = 32
2x2 = 32
x2 = 16
x = 4.
[∵ region lies in first quadrant]
Also, y = 4
Thus, the line (1) and the circle (2) meet each other at B (4,4), in the first quadrant.
Draw BM perp. to x – axis.

∴ Reqd. area = area of the region OMBO + area of the region BMAB …(3)
Now, area of the region OMBO
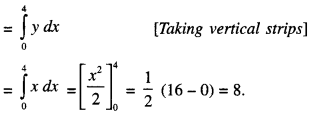
Again, area of the region BMAB
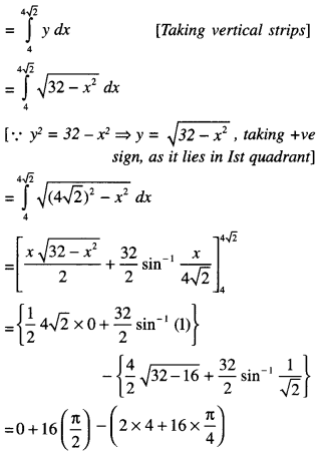
= 8π – (8 + 4π) = 4π – 8
∴ From (3),
Required area = 8 + (4π – 8) = 4π sq. units.
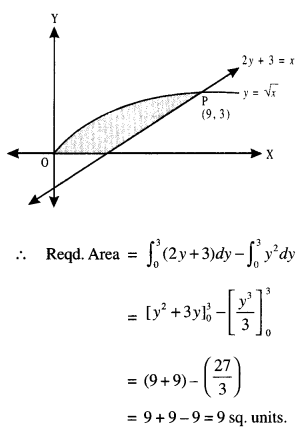
The given curves are
y = √x ………….(1)
and 2y + 3 = x …(2)
Solving (1) and (2), we get;
![]()
Squaring, 2y + 3 = y2
⇒ y22 – 2y – 3 = 0
⇒ (y + 1)(y-3) = 0 ⇒ y = -1, 3
⇒ y = 3 [∵ y > 0]
Putting in (2),
x = 2(3) + 3 = 9.
Thus, (1) and (2) intersects at (9, 3).
The given curves are;
x2 + y2 = 8 ………… (1)
x2 = 2y ………… (2)
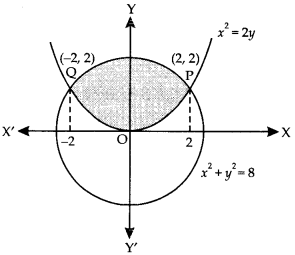
Solving (1) and (2):
8 – y2= 2y
⇒ y2 + 2y – 8 = 0
⇒ (y + 4) (y – 2) = 0
= y = -4,2
⇒ y = 2. [∵ y > 0]
Putting in (2), x2 = 4
⇒ x = -2 or 2.
Thus, (1) and (2) intersect at P(2, 2) and Q(-2, 2).
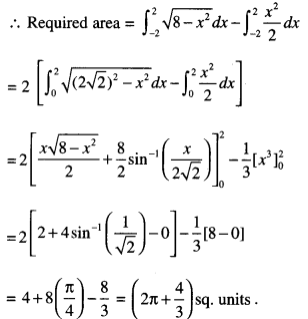
Case Study Answers:
1. Answer :
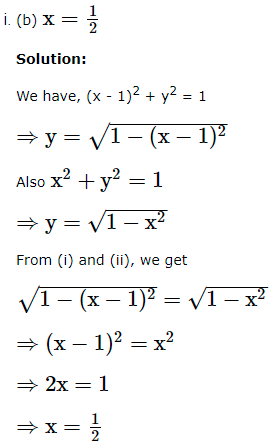
![]()

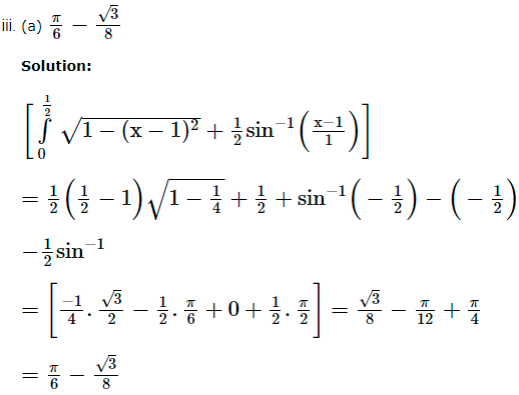
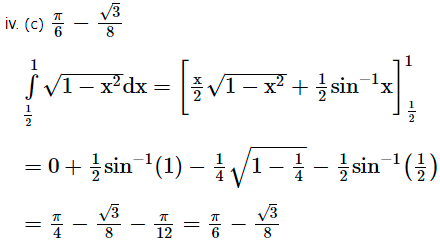

2. Answer :