https://makemynotes.in/probability-2/

PROBABILITY

1.Probability is a quantitative measure of certainty.

2.In the experimental approach to probability, we find the probability of the occurrence of an event by actually performing the experiment a number of times and adequate recording of the happening of event.

3.In the theoretical approach to probability, we try to predict what will happen without actually performing the experiment.

4.The experimental probability of an event approaches to its theoretical probability if the number of trials of an experiment is very large.

5.An outcome is a result of a single trial of an experiment.

6.The word ‘experiment’ means an operation which can produce some well-defined outcome(s).

There are two types of experiments:

i.Deterministic experiments: Experiments which when repeated under identical conditions produce the same results or outcomes are called deterministic experiments.

ii.Random or Probabilistic experiment: If an experiment, when repeated under identical conditions, do not produce the same outcome every time but the outcome in a trial is one of the several possible outcomes, then it is known as a random or probabilistic experiment.

In this chapter, the term experiment will stand for random experiment.

7.The collection of all possible outcomes is called the sample space.

8.An outcome of a random experiment is called an elementary event.

9.An event associated to a random experiment is a compound event if it is obtained by combining two or more elementary events associated to the random experiment.

10.An event A associated to a random experiment is said to occur if any one of the elementary events associated to the event A is an outcome.

11.An elementary event is said to be favourable to a compound event A, if it satisfies the definition of the compound event A. In other words, an elementary event E is favourable to a compound event A, if we say that the event A occurs when E is an outcome of a trial.

12.In an experiment, if two or more events have equal chances to occur or have equal probabilities, then they are called equally likely events.

13.The theoretical probability (also called classical probability) of an event E, written as P (E), is defined as.

14.For two events A and B of an experiment:

If P(A) > P(B) then event A is more likely to occur than event B.

If P(A) = P(B) then events A and B are equally likely to occur.

15.An event is said to be sure event if it always occur whenever the experiment is performed. The probability of sure event is always one. In case of sure event elements are same as the sample space.

16.An event is said to be impossible event if it never occur whenever the experiment is performed. The probability of an impossible event is always zero. Also, the number of favourable outcome is zero for an impossible event.

17.Probability of an event lies between 0 and 1, both inclusive, i.e., 0

\leq

P (A)

\leq

18.If E is an event in a random experiment then the event ’not E’ (denoted by

E - -

) is called the complementary event corresponding to E.

19.The sum of the probabilities of all elementary events of an experiment is 1.

20.For an event E, P(

E - -

) = 1 – P(E) , where the event

E - -

representing ’not E” is the complement of event E.

21.Suits of Playing Card

A pack of playing cards consist of 52 cards which are divided into 4 suits of 13 cards each. Each suit consists of one ace, one king, one queen, one jack and 9 other cards numbered from 2 to 10. Four suits are named as spades, hearts, diamonds and clubs.

22.Face Cards

King, queen and jack are face cards.

A picture containing text, queen Description automatically generated

23.The formula for finding the geometric probability of an event is given by:

Here, ‘measure’ may denote length, area or volume of the region or space.

Important Questions

Multiple Choice questions-

1. If P(A) = $\frac{1}{2}$ , P(B) = 0, then P (A/B) is

(a) 0

(b) $\frac{1}{2}$

(d) 1.

2. If A and B are events such that P (A/B) = P(B/A), then

(a) A ⊂ B but A ≠ B

(b) A = B

(d) P (A) = P (B).

3. The probability of obtaining an even prime number on each die when a pair of dice is rolled is

(a) 0

(b) $\frac{1}{3}$

(d) $\frac{1}{36}$

4. Two events A and B are said to be independent if:

(a) A and B are mutually exclusive

(b) P (A’B’) = [1 – P(A)] [1 – P(B)]

(d) P (A) + P (B) = 1.

5. Probability that A speaks truth is $\frac{4}{5}$ . A coin is tossed. A reports that a head appears. The probability that actually there was head is:

(a) $\frac{4}{5}$

(b) $\frac{1}{2}$

(d) $\frac{2}{5}$

6. If A and B are two events such that A ⊂ B and P (B) ≠ 0, then which of the following is correct

(a) P (A / B) = $\frac{p (B)}{p (A)}$

(b) P (A/B) < P (A)

(d) None of these.

7. If A and B are two events such that P (A) ≠ 0 and P (B/A) = 1, then

(a) A ⊂ B

(b) B ⊂ A

(d) A = ø

8. If P (A/B) > P (A), then which of the following is correct?

(a) P (B/A) < P (B)

(b) P (A ∩ B) < P (A).P(B)

(d) P (B/A) = P (B).

9. If A and B are any two events such that

P (A) + P (B) – P (A and B) = P (A), then:

(a) P (B/A) = 1

(b) P (A/B) = 1

(d) P (A/B) = 0

10. Suppose that two cards are drawn at random from a deck of cards. Let X be the number of aces obtained. What is the value of E (X)?

(a) $\frac{37}{221}$

(b) $\frac{5}{13}$

(d) $\frac{2}{13}$

Very Short Questions:

1.If A and B are two independent event, prove that A’ and B are also independent. (C.B.S.E. Sample Paper 2018-19)

2.One card is drawn from a pack of 52 cards so that each card is equally likely to be se-lected. Prove that the following cases are in-dependent:

• A: “The card drawn is a spade”

B: “The card drawn is an ace.” (N.C.E.R.T.)

• A: “The card drawn is black”

B: “The card drawn is a king.” (.N.C.E.R.T.)

3.A pair of coins is tossed once. Find the probability of showing at least one head.

4.P(A) = 0.6, P(B) = 0. 5 and P(A/B) = 0.3, then find P(A∪ B) (C.B.S.E. Sample Paper 2018-19)

5.One bag contains 3 red and 5 black balls. Another bag contains 6 red and 4 black balls. A ball is transferred from first bag to the second bag and then a ball is drawn from the second bag. Find the probability that the ball drawn is red. (C.B.S.E. Sample Paper 2018-19)

6.Evaluate P(A ∪ B), if 2P(A) = P(B) =

\frac{5}{13}

and P(A/B) =

\frac{2}{5}

(C.B.S.E. 2018 C)

Short Questions:

1.Given that A and B are two independent events such that P(A) = 0.3 and P(B) = 0.5. Find P(A/B). (C.B.S.E. 2019 C)

2.A bag contains 3 white and 2 red balls, another bag contains 4 white and 3 red balls. One ball is drawn at random from each bag.

Find the probability that the balls drawn are one white and one red. (C.B.S.E. 2019 C)

3.The probabilities of A, B and C solving a problem independently are

\frac{1}{2}

\frac{1}{3}

and

\frac{1}{4}

respectively. If all the three try to solve the problem independently, find the probability that the problem is solved. (C.B.S.E. 2019 C)

4.A die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event “number is even” and B be the event “number is marked red”. Find whether the events A and B are independent or not. {Delhi 2019)

5.A die is thrown 6 times. If “getting an odd number” is a success, what is the probability of (i) 5 successes (ii) at most 5 successes? (Delhi 2019)

6.The random variable ‘X’ has a probability distribution P(X) of the following form, where ‘k’ is some number:

Schematic Description automatically generated with medium confidence

Determine the value of ‘P. (Outside Delhi 2019)

7.Out of 8 outstanding students of a school, in which there are 3 boys and 5 girls, a team of 4 students is to be selected for a quiz competition. Find the probability that 2 boy and 2 girls are selected. (Outside Delhi 2019)

8.12 cards numbered 1 to 12 (one number on one card), are placed in a box and mixed up thoroughly. Then a card is drawn at random from the box. If it is known that the number on the drawn card is greater than 5, find the probability that the card bears an odd number. {Outside Delhi 2019)

Long Questions:

1.A black and a red die are rolled together. Find the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4. (C.B.S.E. 2018)

2.Two numbers are selected at random (with-out replacement) from the first five positive integers. Let X denote the larger of the two numbers obtained. Find the mean and variance of X.

3.The probabilities of two students A and B coming to the school in time are

\frac{3}{7}

and

\frac{5}{7}

respectively. Assuming that the events, ‘A coming in time’ and ‘B coming in time’ are independent, find the probability of only one of them coming to the school in time. (A.I.C.B.S.E. 2013)

4.A speaks truth in 80% cases and B speaks truth in 90% cases. In what percentage of cases are they likely to agree with each other in stating the same fact? (C.B.S.E. Sample Paper 2019-20)

Case Study Questions:

1. In an office three employees Vinay, Sonia and Iqbal process incoming copies of a certain form. Vinay process 50% of the forms, Sonia processes 20% and Iqbal the remaining 30% of the forms. Vinay has an error rate of 0.06, Sonia has an error rate of 0.04 and Iqbal has an error rate of 0.03.

A picture containing sky, toy Description automatically generated

Based on the above information, answer the following questions.

(i)The conditional probability that an error is committed in processing given that Sonia processed the form is:

a.0.0210

b.0.04

c.0.47

d.0.06

(ii)The probability that Sonia processed the form and committed an error is:

a.0.005

b.0.006

c.0.008

d.0.68

(iii)The total probability of committing an error in processing the form is:

a.0

b.0.047

c.0.234

d.1

(iv)The manager of the company wants to do a quality check. During inspection he selects a form at random from the days output of processed forms. If the form selected at random has an error, the probability that the form is NOT processed by Vinay is:

(v)Let A be the event of committing an error in processing the form and let E₁, E₂ and E₃ be the events that Vinay, Sonia and Iqbal processed the form. The value of

is:

a.0

b.0.03

c.0.06

d.1

2. Between students of class XII of two schools A and B basketball match is organised. For which, a team from each school is chosen, say T₁ be the team of school A and T₂ be the team of school B. These teams have to play two games against each other. It is assumed that the outcomes of the two games are independent. The probability of T₁ winning, rawmg an osrng a game against T₂ are $\frac{1}{2}$ , $\frac{3}{10}$ and $\frac{1}{5}$ respectively.

Each team gets 2 points for a win, 1 point for a draw and 0 point for a loss in a game.

Let X and Y denote the total points scored by team A and B respectively, after two games.

A group of people playing basketball Description automatically generated with medium confidence

Based on the above information, answer the following questions.

Text Description automatically generated

Text Description automatically generated with medium confidence

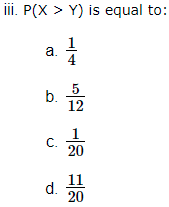
A picture containing schematic Description automatically generated

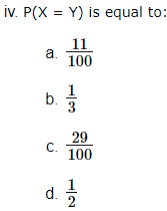
Table Description automatically generated with low confidence

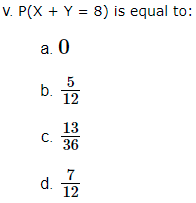
Table Description automatically generated with medium confidence

Answer Key-

Multiple Choice questions-

1.Answer: (c) not defined

2.Answer: (d) P (A) = P (B).

3.Answer: (d)

\frac{1}{36}

4.Answer: (b) P (A’B’) = [1 – P(A)] [1 – P(B)]

5.Answer: (a)

\frac{4}{5}

6.Answer: (c) P (A/B) ≥ P (A)

7.Answer: (a) A ⊂ B

8.Answer: (c) P (B/A) > P (B)

9.Answer: (b) P (A/B) = 1

10.Answer: (d)

\frac{2}{13}

Very Short Answer:

1.Solution:

Since A and B are independent events, [Given]

.-. P (A ∩ B) = P (A) . P(B) …(1)

Now P(A’∩B) = P (B) – P (A ∩ B)

= P(B) – P (A) P( ∩ B) [Using (1)]

= (1 – P(A)) P(B) = P(A’) P(B).

Hence, A’ and B are independent events.

2.Solution:

(a) P(A) = $\frac{13}{52}$ = $\frac{1}{4}$ , P(B) = $\frac{4}{52}$ = $\frac{1}{13}$

P(A∩B) = $\frac{1}{52}$ = $\frac{1}{4}$ ⋅ $\frac{1}{13}$ = p(A).p(B)

Hence, the events A and B are independent

(b) P(A) = $\frac{26}{52}$ = $\frac{1}{2}$ , P(B) = $\frac{4}{52}$ = $\frac{1}{13}$

P(A∩B) = $\frac{2}{52}$ = $\frac{1}{26}$ = $\frac{1}{2}$ ⋅ $\frac{1}{13}$ = P(A) . P(B)

Hence, the events A and B are independent

3.Solution:

S, Sample space = {HH, HT, TH, TT}

where H ≡ Head and T ≡ Tail.

∴ P (at least one head) = $\frac{3}{4}$ .

4.Solution:

We have: P(A/B) = 0.3

$\frac{P (A \cap B)}{P (B)}$ = 0.3

$\frac{P (A \cap B)}{0.5}$ = 0.3

P (A ∩ B) = 0.5 x 0.3 = 0.15.

Now, P(A∪B) = P(A) + P(B) –P(A ∩ B)

= 0.6 + 0.5 – 0. 15

Hence, P (A ∪ B) = 1.1 – 0.15 = 0.95.

5.Solution:

P (Red transferred and red drawn or black transferred red drawn)

Solution:

P(A∪B) = P(A) + P(B) – (A ∩ B)

Short Answer:

1.Solution:

We have:

P(A)= 0.3 and P(B) = 0.5.

Now P (A ∩ B) = P(A). P(B)

[∵A and B are independent events]

= (0.3) (0.5) = 0.15.

2.Solution:

Reqd. probability

= P (White, Red) + P (Red, White)

3.Solution:

A picture containing text Description automatically generated

Probability that the problem is solved

= Probability that the problem is solved by at least one person

4.Solution:

Here, A: number is even i.e.,

A = {2,4,6}

and B: number is red i.e.,

B = {1,2,3}

And,

P (A ∩ B) = P(Number is even and red) = $\frac{1}{6}$ .

Thus, P (A ∩ B) ≠ P(A). P(B)

Hence, the events A and B are not independent.

5.Solution:

Probability of getting an odd number is one 3 1

Probability of getting an even number in one 3

Also, n = 6.

(i) P (5 successes) = P(5) = ⁶C₅ q¹ p⁵

(ii) P (at most 5 successes)

= P (0) + P(1) + … + P(5) = 1 – P(6)

= 1 – ⁶C₆ q⁰ p⁶

6.Solution:

We have: P (X = 0) + P (X = 1) + P (X = 2) = 1

⇒ k + 2k + 3k = 1

⇒ 6k = 1.

7.Solution:

8.Solution:

Let the events be as :

A: Card bears an odd number.

B: Number on the card is greater than 5.

A∩B = {7, 9, 11}.

Long Answer:

1.Solution:

Let the events be as:

E: Sum of numbers is 8

F: Number of red dice less than 4.

E: {(2, 6), (3, 5), (4, 4), (5, 3), (6, 2)}

F = {(1, 1), (2, 1), … (6, 1), (1, 2), (2, 2), … (6, 2), (1, 3), (2, 3), … (6, 2) (6, 3)}

and E ∩ F = {(5, 3), (6, 2)}

2.Solution:

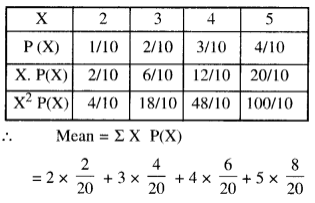
The first five positive integers are 1, 2, 3, 4 and 5.

We select two positive numbers in 5 x 4 = 20 way.

Out of three, two numbers are selected at ran-dom.

Let ‘X’ denote the larger of the two numbers.

X can be 2, 3, 4 or 5.

∴ P (X = 2) = P (Larger number is 2)

{(1, 2), (2,1)} = $\frac{2}{20}$

Similarly, P (X = 3) = $\frac{4}{20}$ ,

P (X = 4) = $\frac{6}{20}$

and P (X = 5) = $\frac{8}{20}$

Hence, the probability distribution is:

Class 12 Maths Important Questions Chapter 13 Probability 2

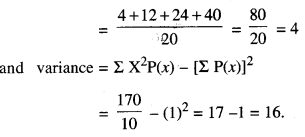
Class 12 Maths Important Questions Chapter 13 Probability 3

3.Solution:

We have: P(A) = Probability of student A coming to school in time = $\frac{3}{7}$

P(B) = Probability of student B coming to school in time = $\frac{5}{7}$

∴ Probability that only one of the students coming to school in time

Text Description automatically generated with medium confidence

4.Solution:

∴ P(Agree) = P(Both speak the truth or both tell a lie)

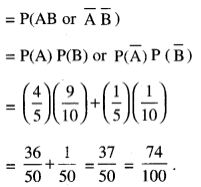
Class 12 Maths Important Questions Chapter 13 Probability 4

Hence, the reqd. percentage = 74%.

Case Study Answers:

1. Answer :
Let A be the event of commiting an error and E₁, E₂ and E₃ be the events that Vinay, Sonia and Iqbal processed the form.

(i)(b) 0.04

Solution:

Required probability = P(A|E₂)\

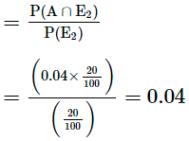
Diagram, text Description automatically generated

(ii)(c) 0.008

Solution:

Required probability = P(A ∩ E2)

(iii)(b) 0.047

Solution:

Total probability is given by

P(A) = P(E₁) × P(A|E₁) + P(E₂) × P(A|E₂) + P(E₃) × P(A|E₃).

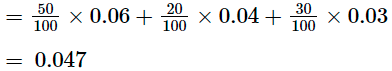
A picture containing logo Description automatically generated

(iv)(d) 17471747

Solution:

Using Bayes’ theorem, we have

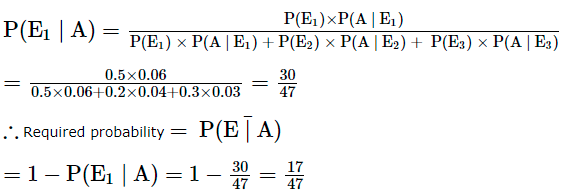
Text, letter Description automatically generated

(v)(d) 1

Solution:

A picture containing diagram Description automatically generated

2. Answer :

(i)(a)

\frac{1}{2}

Solution:

Clearly, P(T₂ winning a match against T₁)

= P(T₁ losing) = $\frac{1}{5}$

(ii)(d)

\frac{3}{10}

Solution:

Clearly, P(T₂ drawing a match against T₁)

= P(T₁ drawing) = $\frac{3}{10}$

(iii)(d)

\frac{11}{20}

Solution:

According to given information, we have the following possibilities for the values of X and Y.

X	4	3	2	1	0
Y	0	1	2	3	4

Now, P(X > Y) = P(X = 4, Y = 0) + P(X = 3, Y = 1)

= P(T₁ win) P(T₁ win) + P(T₁ win) P(match draw) + P(match draw) P(T₁ win)

A screenshot of a computer Description automatically generated with low confidence

(iv)(c)

\frac{29}{100}

Solution:

P(X = Y) = P(X = 2, Y = 2)

= P(T₁ win) P(T₂ win) + P(T₂ win) P(T₁ win) + P(match draw) P(match draw)

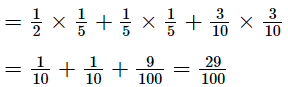
A screenshot of a computer Description automatically generated with low confidence

(v)(a) 0

Solution:

From the given information, it is clear that maximum sum of X and Y can be 4, therefore P(X + Y = 8) = 0.

Leave a Reply Cancel reply

Related Stories

Linear Programming

Three dimensional Geometry

Vector Algebra

You may have missed

বিজ্ঞানের জয়যাত্রা ও মানুষের ভবিষ্যৎ-Bigyaner jayayatra o manuser bhabisyat

বাংলার ঋতুচক্র-Banglar Rituchakra

প্রিয় গ্রন্থকার -“মানিক বন্দ্যোপাধ্যায়”-Priya granthakar- Manik bandyopadhay

তোমার প্রিয় কবি (জীবনানন্দ দাস )-Tomar priya kabi (Jibanananda das)